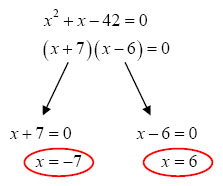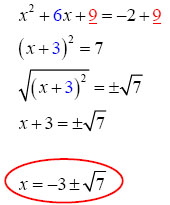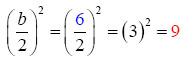How to Solve Quadratic Equations

There are three ways to learn how to solve quadratic equations. Choose your method or scroll down to view all three:

You may also be interested in our quadratic equations solver. You can find it for free at our quadratic formula page, or a larger version here.

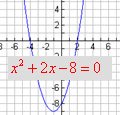
A quadratic equation has a term with x² in it. See the examples below.

A quadratic equation is said to be in standard form whenever the exponents go in descending order. In other words, they fit the pattern...

Now that we have that out of the way, let's solve.

Need more information about factoring? Factoring & Factoring Polynomials.

Once you have factored the polynomial, you will use the zero product property - basically you will set each factor = 0.

To solve by factoring, you will first need to set the quadratic equal to 0. Then, you will factor the equation. For more information, click the links above. After the equation is factored, set each individual factor equal to 0. Then solve the simple equations. Your final answers are x = 6, -7.

Make sure to get your quadratic into standard form (see above), and then plug the values into the quadratic formula.

Completing the square is the process of creating a perfect square trinomial.

Take a look at the example and step by step instructions below of how to solve quadratic equations by completing the square.

Example: x2 + 6x = -2			Add 9: Factor: Square root: Subtract 3:   Final Answer:
Find the amount to add

Congratulations, you just learned three different methods of how to solve quadratic equations.

Return to more free algebra help or visit the GradeA homepage for more free math help.